Researchers discovered how a layer of organic molecules on a nanoparticle surface detaches to create a highly catalytic pocket for reducing CO2 to CO. Read more »![]()
![]()
All News & Updates
Pacific Kelp Forests Are Far Older than We Thought
Researchers scanned newly discovered kelp fossils using x-ray tomography at the ALS. The images provided morphological information about the ancient kelp and, along with isotopic analyses, provided insights into the evolutionary history of northeastern Pacific Ocean kelp forests, which flourished more than 32 million years ago. Read more »
Submit Nominations for 2024 User Meeting Awards
Help us recognize members of the ALS community by submitting nominations for the Shirley Award, Halbach Award, Renner Award, and new for this year: Early Career Award. Deadline: May 24. Read more »
Jillian Hiu, Mechanical Engineering Associate
Growing up in San Jose, Jillian Hiu heard about Berkeley Lab. Now, she’s part of the research mission here, working on components for the QERLIN beamline. Find out how she combines science and art, and how her pet surprised her. Read more »
Surtsey Volcano: A Rare Window into Earth’s Oceanic Crust
Surtsey, a very young oceanic island in Iceland, emerged through explosive volcanic activity in 1963. Utilizing various techniques, including x-ray microdiffraction at the ALS, researchers gained unique insights into the transformation of volcanic glass to form mineral cements in the basaltic rock of underwater volcanoes. Read more »
Vote: ALS Users’ Association Charter Change
The UEC is recommending establishing an ALS Early Career Award, to be presented for the first time at the upcoming User Meeting, to recognize significant scientific contributions from early-career investigators who have performed original and independent research at the ALS. To institute this award, a change must be made to the users’ association charter. Read more »
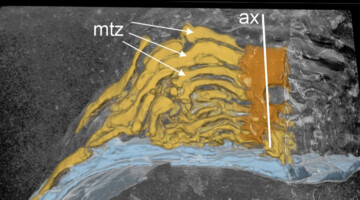
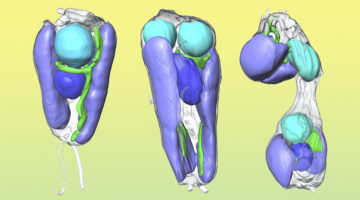
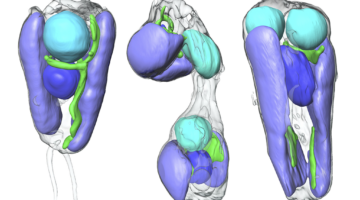
Symbiotic Nitrogen-Fixing Microbe Evolves into Organelle
Researchers found that a symbiont capable of fixing nitrogen (turning it into a biologically usable form) has evolved into an organelle—an intrinsic part of the algae cells that host it. The discovery is of great interest for understanding organelle genesis and for efforts to engineer agricultural plants with built-in nitrogen-fixing capabilities. Read more »![]()
![]()
New Insights Lead to Better Next-Gen Solar Cells
Perovskites show great promise to reduce the costs of solar power but are not yet durable enough to be commercially viable. Researchers used simultaneous characterization techniques to understand why a simplified fabrication process works so well, providing key insights to nudge perovskites closer to commercialization. Read more »
Scientists Discover First Nitrogen-Fixing Organelle
In two recent papers, an international team of scientists describe the first known nitrogen-fixing organelle within a eukaryotic cell. The organelle is the fourth example in history of primary endosymbiosis—the process by which a prokaryotic cell is engulfed by a eukaryotic cell and evolves beyond symbiosis into an organelle. Read more »
Shedding Light on Sea Creatures’ Secrets
Exactly how does coral make its skeleton, a sea urchin grow a spine, or an abalone form the mother-of-pearl in its shell? A new study at the ALS revealed that this process of biomineralization, which sea creatures use to lock carbon away in their bodies, is more complex and diverse than previously thought. Read more »
- 1
- 2
- 3
- …
- 123
- Next Page »